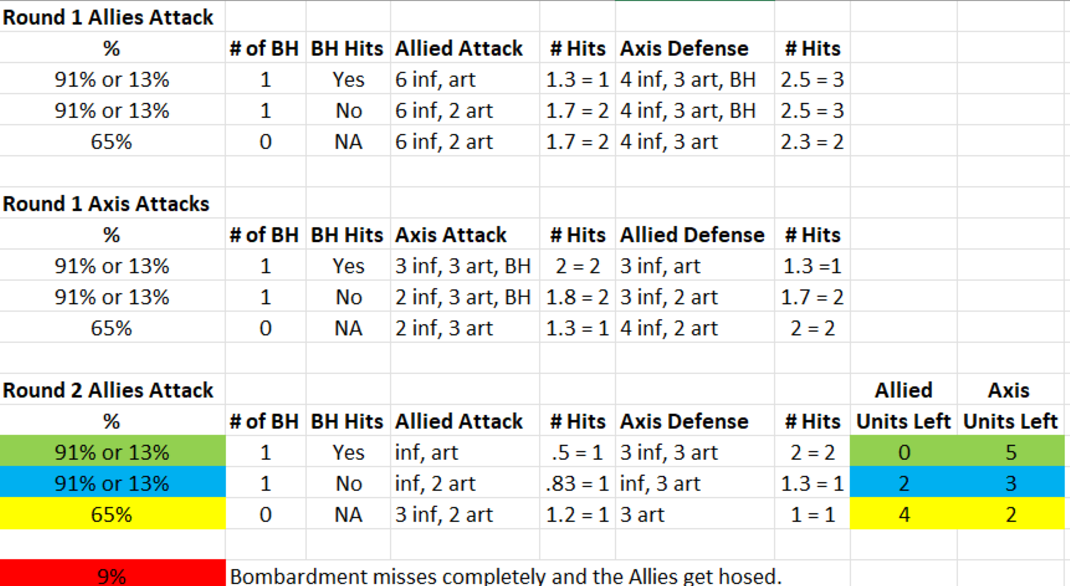
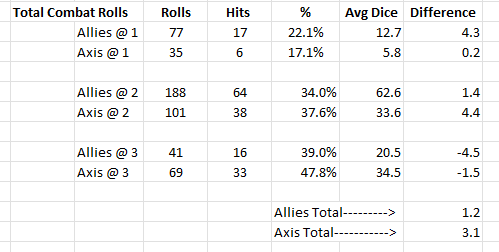
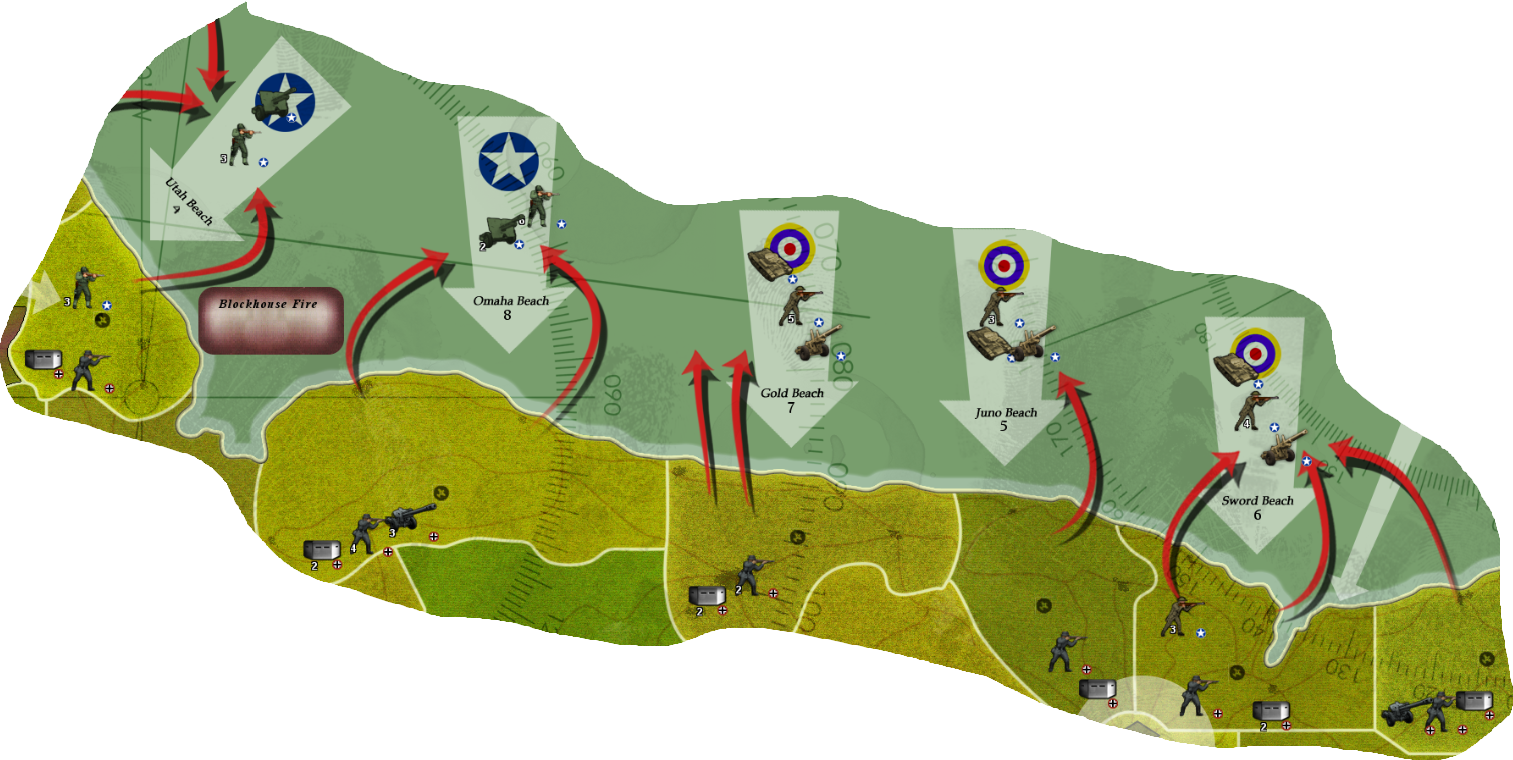
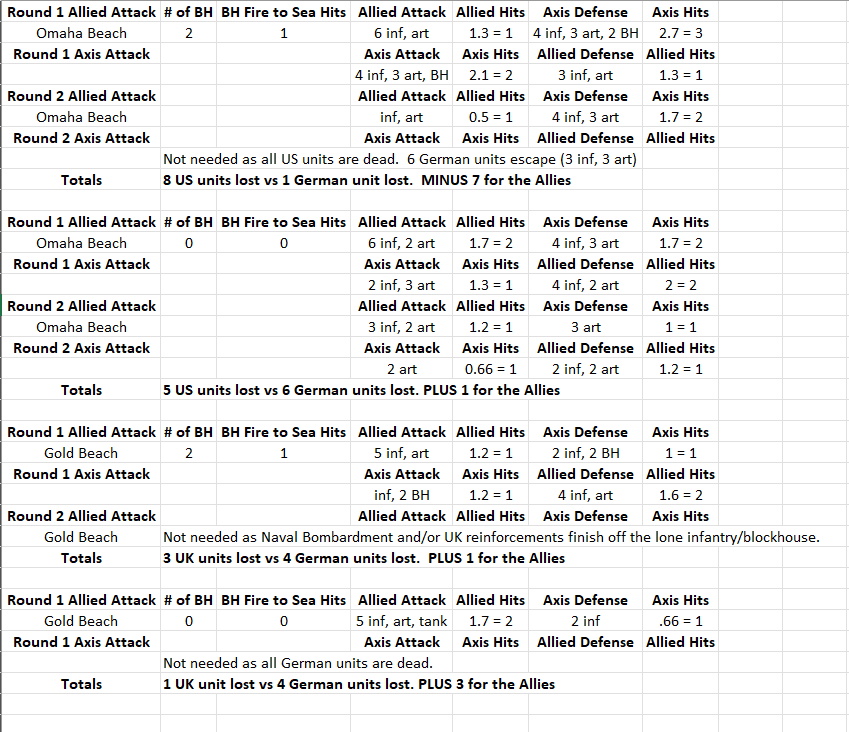
TripleA Turn Summary for game: World War II v5 1942 SE TR
Game History
Round: 1 Purchase Units - British British buy 3 fighters; Remaining resources: 1 PUs; Combat Move - British 1 destroyer moved from 10 Sea Zone to 7 Sea Zone 1 fighter moved from United Kingdom to 7 Sea Zone 1 fighter moved from United Kingdom to 3 Sea Zone 1 bomber moved from United Kingdom to 7 Sea Zone 1 fighter moved from Egypt to 37 Sea Zone 1 carrier, 1 cruiser and 1 fighter moved from 35 Sea Zone to 37 Sea Zone 2 infantry moved from India to 35 Sea Zone 2 infantry and 1 transport moved from 35 Sea Zone to 37 Sea Zone 2 infantry moved from 37 Sea Zone to East Indies 2 infantry moved from Eastern Australia to 39 Sea Zone 1 cruiser, 2 infantry, 1 submarine and 1 transport moved from 39 Sea Zone to 37 Sea Zone 2 infantry moved from 37 Sea Zone to East Indies 1 armour, 1 artillery and 1 infantry moved from Egypt to Trans-Jordan 1 infantry moved from Persia to Trans-Jordan Combat - British Battle in 3 Sea Zone British attack with 1 fighter Germans defend with 1 transport 1 transport owned by the Germans lost in 3 Sea Zone British win with 1 fighter remaining. Battle score for attacker is 7 Casualties for Germans: 1 transport Battle in 37 Sea Zone British attack with 1 carrier, 2 cruisers, 2 fighters, 1 submarine and 2 transports Japanese defend with 1 battleship, 1 carrier and 2 fighters British roll dice for 1 submarine in 37 Sea Zone, round 2 : 0/1 hits, 0.33 expected hits British roll dice for 1 carrier, 2 cruisers, 2 fighters and 2 transports in 37 Sea Zone, round 2 : 1/5 hits, 2.17 expected hits Units damaged: 1 battleship owned by the Japanese Japanese roll dice for 2 fighters in 37 Sea Zone, round 2 : 2/2 hits, 1.33 expected hits Japanese roll dice for 1 battleship and 1 carrier in 37 Sea Zone, round 2 : 1/2 hits, 1.00 expected hits 1 cruiser owned by the British, 1 fighter owned by the British and 1 carrier owned by the British lost in 37 Sea Zone British roll dice for 1 submarine in 37 Sea Zone, round 3 : 0/1 hits, 0.33 expected hits British roll dice for 1 cruiser, 1 fighter and 2 transports in 37 Sea Zone, round 3 : 2/2 hits, 1.00 expected hits Japanese roll dice for 2 fighters in 37 Sea Zone, round 3 : 2/2 hits, 1.33 expected hits Japanese roll dice for 1 battleship and 1 carrier in 37 Sea Zone, round 3 : 0/2 hits, 1.00 expected hits 1 cruiser owned by the British and 1 fighter owned by the British lost in 37 Sea Zone 1 fighter owned by the Japanese and 1 carrier owned by the Japanese lost in 37 Sea Zone British roll dice for 1 submarine in 37 Sea Zone, round 4 : 0/1 hits, 0.33 expected hits British roll dice for 2 transports in 37 Sea Zone, round 4 : 0/0 hits, 0.00 expected hits Japanese roll dice for 1 fighter in 37 Sea Zone, round 4 : 1/1 hits, 0.67 expected hits Japanese roll dice for 1 battleship in 37 Sea Zone, round 4 : 0/1 hits, 0.67 expected hits 1 transport owned by the British and 2 infantry owned by the British lost in 37 Sea Zone British roll dice for 1 submarine in 37 Sea Zone, round 5 : 0/1 hits, 0.33 expected hits British roll dice for 1 transport in 37 Sea Zone, round 5 : 0/0 hits, 0.00 expected hits Japanese roll dice for 1 fighter in 37 Sea Zone, round 5 : 0/1 hits, 0.67 expected hits Japanese roll dice for 1 battleship in 37 Sea Zone, round 5 : 1/1 hits, 0.67 expected hits 1 submarine owned by the British lost in 37 Sea Zone 1 transport owned by the British retreated to 38 Sea Zone Japanese win with 1 battleship and 1 fighter remaining. Battle score for attacker is -53 Casualties for British: 1 carrier, 2 cruisers, 2 fighters, 2 infantry, 1 submarine and 1 transport Casualties for Japanese: 1 carrier and 1 fighter Turning on Edit Mode EDIT: Turning off Edit Mode Battle in Trans-Jordan British attack with 1 armour, 1 artillery and 2 infantry Germans defend with 1 artillery British roll dice for 1 armour, 1 artillery and 2 infantry in Trans-Jordan, round 2 : 1/4 hits, 1.33 expected hits Germans roll dice for 1 artillery in Trans-Jordan, round 2 : 0/1 hits, 0.33 expected hits 1 artillery owned by the Germans lost in Trans-Jordan British win, taking Trans-Jordan from Germans with 1 armour, 1 artillery and 2 infantry remaining. Battle score for attacker is 4 Casualties for Germans: 1 artillery Battle in 7 Sea Zone British attack with 1 bomber, 1 destroyer and 1 fighter; Russians loiter and taunt Germans defend with 1 cruiser and 1 submarine British roll dice for 1 bomber, 1 destroyer and 1 fighter in 7 Sea Zone, round 2 : 2/3 hits, 1.50 expected hits Germans roll dice for 1 submarine in 7 Sea Zone, round 2 : 0/1 hits, 0.17 expected hits Germans roll dice for 1 cruiser in 7 Sea Zone, round 2 : 1/1 hits, 0.50 expected hits 1 destroyer owned by the British lost in 7 Sea Zone 1 submarine owned by the Germans and 1 cruiser owned by the Germans lost in 7 Sea Zone British win with 1 bomber and 1 fighter remaining. Battle score for attacker is 10 Casualties for British: 1 destroyer Casualties for Germans: 1 cruiser and 1 submarine 1 fighter owned by the Japanese forced to land in East Indies Turning on Edit Mode EDIT: Turning off Edit Mode Turning on Edit Mode EDIT: Adding units owned by British to 37 Sea Zone: 2 infantry and 1 transport EDIT: Adding units owned by Japanese to 37 Sea Zone: 1 carrier and 2 fighters EDIT: Removing units owned by Japanese from East Indies: 1 fighter EDIT: Adding units owned by British to 37 Sea Zone: 1 carrier and 1 fighter EDIT: Turning off Edit Mode Turning on Edit Mode EDIT: Adding units owned by British to 31 Sea Zone: 1 cruiser EDIT: Turning off Edit Mode EDIT: 2 infantry and 1 transport moved from 37 Sea Zone to 35 Sea Zone EDIT: 2 infantry and 1 transport moved from 38 Sea Zone to 35 Sea Zone EDIT: British undo move 2. EDIT: British undo move 1. EDIT: 2 infantry and 1 transport moved from 38 Sea Zone to 35 Sea Zone 1 carrier, 1 fighter, 2 infantry and 1 transport moved from 37 Sea Zone to 35 Sea Zone 1 cruiser moved from 31 Sea Zone to 35 Sea Zone 1 infantry moved from India to Persia 1 infantry moved from Burma to India 1 infantry moved from Union of South Africa to Rhodesia 1 infantry moved from Western Australia to Eastern Australia 1 infantry moved from Western Canada to Eastern Canada 1 bomber moved from 7 Sea Zone to Caucasus 1 fighter moved from 7 Sea Zone to Archangel 1 fighter moved from 3 Sea Zone to Archangel Place Units - British Turning on Edit Mode EDIT: Removing units owned by British from 35 Sea Zone: 1 fighter EDIT: Adding units owned by British to 35 Sea Zone: 1 cruiser EDIT: Turning off Edit Mode 2 fighters placed in 35 Sea Zone 1 fighter placed in India Turn Complete - British British collect 31 PUs; end with 32 PUs 1 unit repaired.Combat Hit Differential Summary :
Japanese regular : -0.33 Germans regular : 0.00 British regular : -1.33